Basic properties and ideas about Rhombus

In a two dimensional geometry, a rhombus is a quadrilateral where all of the four sides are equivalent. Thus, we can say that it is a symmetrical or equilateral quadrilateral. Plural type of rhombus is rhombi and rhombuses. The rhombus in mathematics is otherwise called Diamond, we can see this precious stone shape on playing a game of cards.

Definition of Rhombus

Rhombus is a kind of quadrilateral whose opposite sides are equal and each of the four sides is equivalent in length. In addition, the rhombus is otherwise called diamond. The rhombus in mathematics is an extraordinary instance of parallelogram and kite. On the off chance that the angles of the rhombus are right angles or 90°, the rhombus is known as square.

Different Properties of Angles in a Rhombus

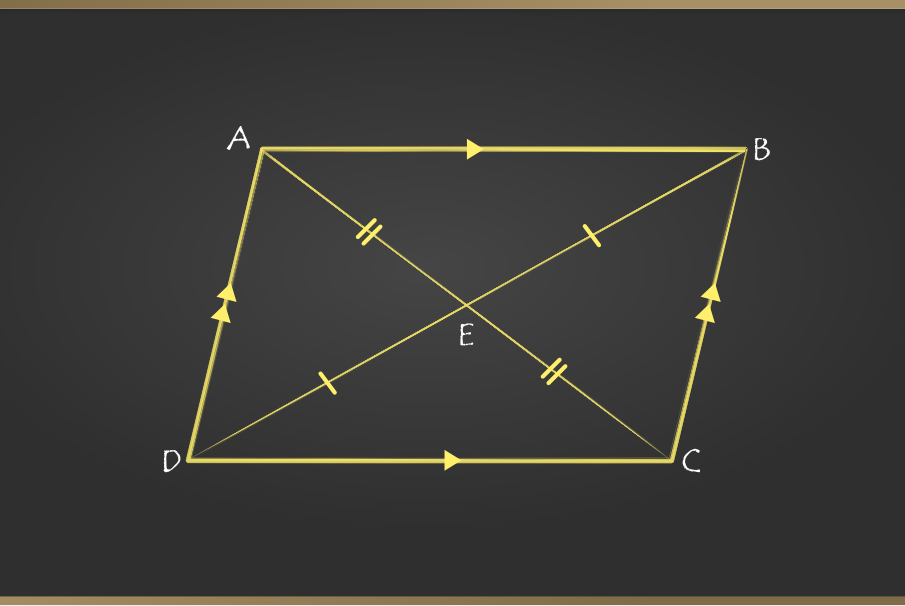
Let us assume a rhombus ABCD. We see here that, AB, BC, DC and AD are the sides of the rhombus.

A few significant properties of angles of rhombus in mathematics are given beneath:

• A rhombus has 4 inside angles.
• Amount of all inside angles of the rhombus is 360 degrees.
• Diagonal of rhombus divides the angle of rhombus in exact halves.
• Inverse angles of the rhombus are equivalent.
• Adjacent angles of rhombus are supplementary sets.

Relation of Rhombus and Square

Indeed, we can say a square is a rhombus yet all rhombuses can’t be a square.

How might we say a square is a rhombus?

We say that because of some resemblance among square and rhombus which are given underneath:

• In a square, lengths of all sides of square are equal whereas in a rhombus, all sides of rhombus are equivalent in length.
• Diagonals of square separate each other in equal halves whereas Diagonals of rhombus also divides each other in equal halves.
• Adjacent angle of square are supplementary, similarly Adjacent angle of rhombus are supplementary pair.
• The opposite angles of square are equal and eventually, opposite angle of rhombus are equivalent

Significant Equations and Formulae of Rhombus

Area of Rhombus: We can characterize the area of a rhombus in mathematics as how much space covered by each of the four sides of a rhombus in a two layered plane.

The area of rhombus by utilizing diagonals

The area of the rhombus is the half of the product of the length of the diagonals of the rhombus.

Thus, the area of rhombus can be described as: (D1 * D2)/2. Where,D1 and D2 are the length of the diagonals of rhombus.

The area of rhombus in mathematics by utilizing elevation or height and base of rhombus

The area of a rhombus is the product of the length of base and elevation of the rhombus. It can be justified with a perpendicular distance from base to the inverse side of rhombus.

The area of rhombus by utilizing elevation and base of rhombus

The area of rhombus = (B * H), where B and H are length of base and length of elevation of rhombus, individually.

The area of rhombus by utilizing trigonometric functions and length

The area of rhombus is the product of the square of length of one side of the rhombus in mathematics and sine of an interior angle of the rhombus.

Let us revise the different properties of rhombus altogether.

Significant Properties of Rhombus

Significant properties which are trailed by rhombus in mathematics are given underneath:

• All sides of the rhombus are equivalent.
• Inverse sides of rhombus are parallel to one another.
• Diagonals of rhombus divide each other at right angles or 90°.
• Diagonals of the rhombus divide the rhombus in four compatible triangles.
• We can’t engrave a rhombus within a circle.
• We can’t encircle a circle around the rhombus.
• We get a rectangular shape on the off chance that we join each of the 4 midpoints of the sides of the rhombus and the area of the rectangle would comprise half of the area of the rhombus.

For further concepts on mathematics, please check into Cuemath available online.